1. Phonon angular momentum and its applications
The Einstein-de Haas experiment has provided an effective method in measurements of the gyromagnetic ratio for various materials. The accuracy of gyromagnetic ratio plays an important role in the area of magnetic data recording, and has attracted wide researches in the dynamics, anisotropies, quenching of spin and orbital moments in magnetic materials. In the Einstein-de Haas effect, the phonon angular momentum is taken to be zero. However, we found that the phonon angular momentum can be comparable to the orbital angular momentum of electrons in some magnetic materials. Therefore the past measurements on gyromagnetic ratio need to be corrected by phonon angular momentum. In materials with hexagonal lattices, we found that the intravalley and intervalley scattering of electrons can involve chiral phonons at Brillouin-zone center and corners, respectively. The chiral phonons, which have phonon circular polarization with the same form of phonon angular momentum.
References:
1. Lifa Zhang and Qian Niu, ``Angular Momentum of Phonons and Einstein-de Haas Effect’’, Phys. Rev. Lett. 112, 085503 (2014).
2. Lifa Zhang and Qian Niu, ``Chiral Phonons at High-Symmetry Points in Monolayer Hexagonal Lattices’’, Phys. Rev. Lett. 115, 115502 (2015).
2. Phonon and magnon Hall effects
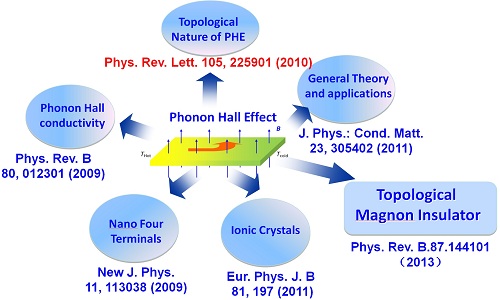
Phonon (magnon) Hall effect, a marriage between phononics (magnonoics) and Hall effect, is opening the door for controlling phonons (magnons) by a magnetic field. Traditionally we thought that we cannot manipulate the phonons (magnons) by the external magnetic field because of the absence of Lorentz force. Therefore it is a big surprise of the experimental finding of phonon and magnon Hall effect. We have successfully proposed two exact and systematic theories for the phonon Hall effect (PHE) in finite sample with heat baths, where we uncover the underlying physical mechanism of the PHE and excavate a serial luxuriant properties of the PHE, such as symmetry criterion, nonlinear dependence on a magnetic field, topological nature and phase transition. Both magnon and phonon belong to quasi-particles of Bosons. After understanding of phonon Hall effect, we study the magnon Hall effect and found the topological magnon insulator in insulating ferromagnet.
3. Interfacial Thermal transport and Rectification
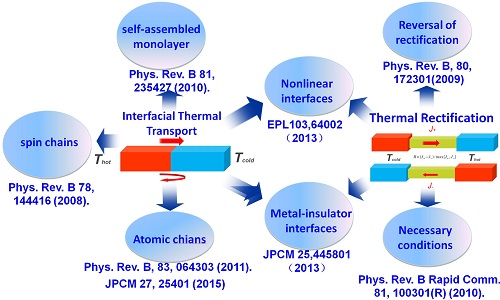
With increasing the density of integration, it becomes more important to dissipate and manage heat in modern electronic devices, which has been recognized to be a key issue in the application of nanoscale thermal transport. In the nanoscale structure, efficient heat removal requires new materials and engineered contacts with high thermal conductance and low thermal interface/contact resistance, urging the studies aimed at exploring such material systems and the thermal transport at interface. To efficient control thermal transport, the thermal rectification is one of most important phenomenon, where heat only transfer along one direction. We found necessary conditions for thermal rectification: phonon incoherence and asymmetry.